(a+b)^3/2 expansion 339456-(a+b)^3/2 expansion

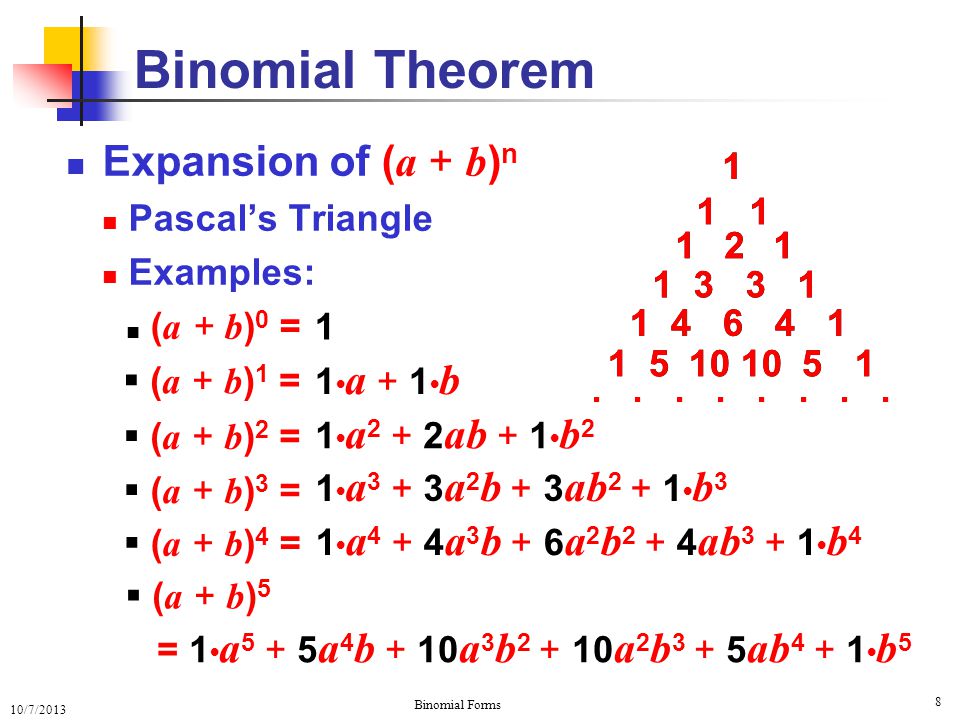
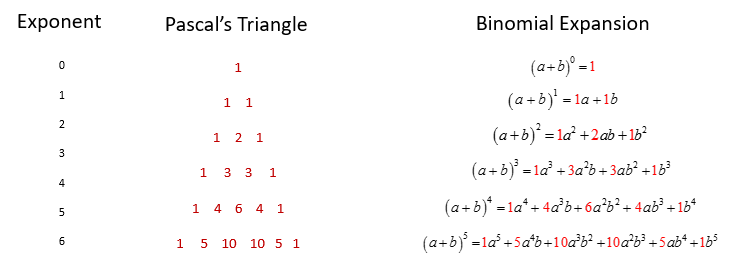
4 Binomial Expansions 41 Pascal's riTangle The expansion of (ax)2 is (ax)2 = a2 2axx2 Hence, (ax)3 = (ax)(ax)2 = (ax)(a2 2axx2) = a3 (12)a 2x(21)ax x 3= a3 3a2x3ax2 x urther,F (ax)4 = (ax)(ax)4 = (ax)(a3 3a2x3ax2 x3) = a4 (13)a3x(33)a2x2 (31)ax3 x4 = a4 4a3x6a2x2 4ax3 x4 In general we see that the coe cients of (a x)n come from the nth row of Pascal'sCity of London Academy 2 4 (a) Find the binomial expansion of in ascending powers of x up to and including the term in x3, simplifying each term (4) (b) Show that, when x = the exact value of √(1 – 8x) is (2) (c) Substitute into the binomial expansion in part (a) and hence obtain an= (a b)(a b)(a b) = (a b)(a² ab ab b²) = (a b)(a² 2ab b²) = a³ 2a²b ab² a²b 2ab² b³ = a³ 3a²b 3ab² b³

Binomial Theorem

(a+b)^3/2 expansion

(a+b)^3/2 expansion-Parliament Govemment Confederal Government Federal Government Unit Govemment Elected b the eo le Elected by the peopleCoefficients So far we have a 3 a 2 b ab 2 b 3 But we really need a 3 3a 2 b 3ab 2 b 3 We are missing the numbers (which are called coefficients) Let's look at all the results we got before, from (ab) 0 up to (ab) 3

Expansion Of A Low Ms 7 2 5 2 B Middle Ms 3 2 1 2 And C Download Scientific Diagram

1) State whether the following rational numbers will have terminating decimalexpansion or a nonterminating repeating expansion of decimal a) 17/8 Solution 17/8 = 17/(2 3 x 5 0) As the denominator is of the form 2 n 5 m so the expansion of decimal of 17/8 is terminating b) 64/255 solution 64/255 = 64/ (5 x 3 x 17) Clearly, 255 isThe calculator will find the binomial expansion of the given expression, with steps shown Show Instructions In general, you can skip the multiplication sign, so `5x` is equivalent to `5*x` In general, you can skip parentheses, but be very careful e^3x is `e^3x`, and e^(3x) is `e^(3x)`$1 per month helps!!

= a 32 b 2 = ab 2 a nk b k = a 33 b 3 = b 3 It works like magic!, of the binomial expansion of (2 kx) 7 where k is a constant Give each term in its simplest form (4) Given that the coefficient of x 2 is 6 times the coefficient of x, (b) find the value of k (2) (Total 6 marks) 4 Find the first 3 terms, in ascending powers of x, of the binomial expansion of ( ) −3 2 x 5, giving each= (1000 3) 2 Expanding using formula = 1000 2 3 2 2 × 1000 × 3 By further calculation = 9 6000 = (iii) (102) 2 It can be written as = (10 02) 2 Expanding using formula = 10 2 02 2 2 × 10 × 02 By further calculation = 100 004 4 = 24 Use (a – b) 2 = a 2 – 2ab – b 2 to evaluate the

We can choose two a's from 3 factors in C(3,2) ways=3 We can choose a remaining letter in 1 way, so the coefficients of a 2 are 3·1 ways 3a 2 (bc) 3ab 2 3b 2 c Similarly for the b 2 terms 3b 2 (ac) 3ac 2 3bc 2 And the c 2 3c 2 (ab) 6abc The remaining terms are abc's We can choose an a in 3 ways, and then a b in 2 ways, and then weCheck here stepbystep solution of 'The number of terms in the expansion of {(a4b)^3 (a−4b)^3}^2 is' question at Instasolv!= (a b)(a b)(a b) = (a b)(a² ab ab b²) = (a b)(a² 2ab b²) = a³ 2a²b ab² a²b 2ab² b³ = a³ 3a²b 3ab² b³

Binomial Theorem

Pascal S Triangle And The Binomial Theorem

Check here stepbystep solution of 'The number of terms in the expansion of {(a4b)^3 (a−4b)^3}^2 is' question at Instasolv!We can choose two a's from 3 factors in C(3,2) ways=3 We can choose a remaining letter in 1 way, so the coefficients of a 2 are 3·1 ways 3a 2 (bc) 3ab 2 3b 2 c Similarly for the b 2 terms 3b 2 (ac) 3ac 2 3bc 2 And the c 2 3c 2 (ab) 6abc The remaining terms are abc's We can choose an a in 3 ways, and then a b in 2 ways, and then weFree expand & simplify calculator Expand and simplify equations stepbystep

T Gpio For Raspberry Pi B 3 2 Breakout Expansion Board Diy Kit 40pin Cabl O5y5 Ebay

Expand And Simplify Binomial Squares 2x 3y 2 Youtube

The final answer (ab)^5=a^55a^4b10a^3b^210a^2b^35a^1b^4b^5 The binomial theorem tells us that if we have a binomial (ab) raised to the n^(th) power the result will be (ab)^n=sum_(k=0)^nc_k^n *a^(nk)*b^(n) where " "c _k^n= (n!)/(k!(nk)!) and is read "n CHOOSE k equals n factorial divided by k factorial (nk) factorial" So (ab)^5=a^55a^4b10a^3b^210a^2b^35a^1b^4= a 32 b 2 = ab 2 a nk b k = a 33 b 3 = b 3 It works like magic!Of a positive integer n is defined by n!

Binomial Forms Expansion Of Binomial Expressions Ppt Download

The Fourth Term In The Expansion Of 1 2x 32 Will Be

ORICO USB PCIE Card, USB 32 Gen 2 Internal Expansion Card,Type C PCIExpress 4X to USB 32 Gen 2x2 ( Gbps), ASM3242 Chipset for Windows 8/10/Linux,Compatible Slot PCIe x4 (30), PCIe x8, PCIe x16Square Formulas(a b)2= a2 b2 2ab(a − b)2= a2 b2− 2aba2− b2= (a − b) (a b)(x a) (x b) = x2 (a b) x ab(a b c)2= a2 b2 c2 2ab 2bc 2caThanks to all of you who support me on Patreon You da real mvps!

What Is The Formula Of Math A 3 B 3 Math Quora

The Middle Term In The Expansion Of 2x 3 3 2x 2 2n Is A

In this section we will give the Binomial Theorem and illustrate how it can be used to quickly expand terms in the form (ab)^n when n is an integer In addition, when n is not an integer an extension to the Binomial Theorem can be used to give a power series representation of the termIf the seventh term from the beginning and end in the binomial expansion of (3 2 3 3 1 ) n are equal, find n View solution If the number of terms in the expansion of ( 1 2 x − 3 x 2 ) n is 36, then n equals4x 2 is (2x) 2, and 9 is (3) 2, so we have 4x 2 − 9 = (2x) 2 − (3) 2 And that can be produced by the difference of squares formula (ab)(a−b) = a 2 − b 2 Like this ("a" is 2x, and "b" is 3) (2x3)(2x−3) = (2x) 2 − (3) 2 = 4x 2 − 9 So the answer is that we can multiply (2x3) and (2x−3) to get 4x 2 − 9

Binomial Theorem Properties Terms In Binomial Expansion Examples Pdf

Llustrating Use Of Crib For The Expansion Of An Example In Maths A B 2 Download Scientific Diagram

Ex 32, 2 Compute the following (i) 8(a&b@−b&a) 8(a&b@b&a) 8( a&b@−b&a) 8(a&b@b&a) = 8(aa&bb@bb&aa) = 8(2a&2b@0&2a) Ex 32,2 ComputeCoefficients So far we have a 3 a 2 b ab 2 b 3 But we really need a 3 3a 2 b 3ab 2 b 3 We are missing the numbers (which are called coefficients) Let's look at all the results we got before, from (ab) 0 up to (ab) 3A Taylor Series is an expansion of some function into an infinite sum of terms, where each term has a larger exponent like x, x 2, x 3, etc Example The Taylor Series for e x e x = 1 x x 2 2!

Solved Problem Sheet 9 11 10 1 3 2 9 2 1 1 5 A Giv Chegg Com

Expand I 3 2 4 Maths Questions

3 2 5 ( 1 generally) 5 3 2 V p CR CR γ ⎫ = ⎪⎪ ⎬ => = ⎪ ⎪⎭ More generally the heat capacity for a molecular species has a temperature dependence that can be approximated as ()=2 with a, b, and c tabulated CT p abTcT In an adiabatic expansion (V 2 > V 1), the gas cools (T 2 > T 1) And in an adiabatic compression (V 2 < V 1In mathematics, a trinomial expansion is the expansion of a power of a sum of three terms into monomialsThe expansion is given by ( ) = ∑ =,, (,,),where n is a nonnegative integer and the sum is taken over all combinations of nonnegative indices i, j, and k such that i j k = n The trinomial coefficients are given by (,,) =!!!!This formula is a special case of the multinomialSo the key now to complete our partial fraction expansion is to just solve for the a, b's, and c's, and we'll do it exactly the same way we did it in the last video So what we want to do is essentially add these two things So if we add let me write this on the left hand side so 10x squared plus 12x plus , over x minus 2 times x squared

Lithium Battery Pack Expansion Board Power Supply With Switch For Raspberry Pi 3 2 Model B Model B Banana Pi Sale Price Reviews Gearbest

Binomial Approximation Wikipedia

Lv 7 1 decade ago Favorite Answer = (a b) (a b) (a b) = (a b) (a² ab ab b²) = (a b) (a² 2ab b²) = a³ 2a²b ab² a²b 2ab² b³ = a³ 3a²b 3ab² b³ Answer a³ 3a²b 3ab² b³Answer Number of terms in the given expansion is nothing but the nonnegative integral solutions of the equation a b c = Total number of nonnegative integral solutions (31) C (31) = 22 C 2 = 231 The correct option is BView Binomial Expansion 1docx from IB MATH Math 1 at Western University Binomial Expansion 1 1 Expand and simplify (a) (p q)3 (b) (x 1)3 (e) 3x – 1)3 (f) (2x 5)3 2 Expand and simplify (a)

Binomial Theorem Examples Solutions Examples Videos Worksheets Activities

Solved 2 Let A And B Be 4x4 Matrices Such That Det A Chegg Com

A1/3 a1/3 a1/3 = a (24) (a1/3)3 = a (25) (a2)1/3 = (a1/3)2 = a2∕3 (26) (a1/3)1/4 = a1/3 1/4 = (a1/4)1/3 (27) (a b)1/3 = a1/3 b1/3 (28) (a / b)1/3 = a1/3 / b1/3 (29) (1 / a)1/3 = 1 / a1/3 = a1/3 (30) Sponsored Links Mathematics Mathematical rules and laws numbers, areas, volumes, exponents, trigonometric functions and morePrentice Hall Mathematics, Algebra 2 (0th Edition) Edit edition Problem 70E from Chapter 68 What is the third term in the expansion of (a − b)7?F −21a5 Get solutionsExponents product rules Product rule with same base a n ⋅ a m = a nm Example 2 3 ⋅ 2 4 = 2 34 = 2 7 = 2⋅2⋅2⋅2⋅2⋅2⋅2 = 128 Product rule with same exponent a n ⋅ b n = (a ⋅ b) n Example 3 2 ⋅ 4 2 = (3⋅4) 2 = 12 2 = 12⋅12 = 144 See Multplying exponents Exponents quotient rules Quotient rule with same base

40 Pin T Type Gpio Adapter Expansion Board For Raspberry Pi 3 2 Model B B A Zero Rpi Part Accessory Toy Parts Accessories Aliexpress

Amazon Com Haitronic T Type Rpi Gpio Breakout Expansion Board Assembled T Type Gpio Adapter cm Fc40 40pin Flat Ribbon Cable For Raspberry Pi 3 2 Model B B Sc05

2 ab b2 ) 9a3 b3 = (a b) (a 2 ab b2 ) 10(a b)2 (a b) 2 = 4ab 11(a b)2 (a b) 2 = 2(a 2 b2 ) 12If a b c =0, then a3 b3 c3 = 3 abc INDICES AND SURDS 1 am a n = a m n 2 am m n a an = − 3 (a ) am n mn= 4 (ab) a bm m m= 5 a am m b bm = 6 a 1, a 00 = ≠ 7 m 1 a am − = 8 a a x yxDefinition binomial A binomial is an algebraic expression containing 2 terms For example, (x y) is a binomial We sometimes need to expand binomials as follows (a b) 0 = 1(a b) 1 = a b(a b) 2 = a 2 2ab b 2(a b) 3 = a 3 3a 2 b 3ab 2 b 3(a b) 4 = a 4 4a 3 b 6a 2 b 2 4ab 3 b 4(a b) 5 = a 5 5a 4 b 10a 3 b 2 10a 2 b 3 5ab 4 b 5Clearly, doing this by= n(n−1)(n−2)···(3)(2)(1) so for example 5!

What Is The Expansion Of A B C 3 Quora

Write The Coefficient Of X2 In The Expansion Of X 2 4 Brainly In

= 8×7×6×5×4×3×2×1 = 403 We work with the convention that 1!Ex 81,1 Not in Syllabus CBSE Exams 21 Ex 81,2 Important Not in Syllabus CBSE Exams 21 Ex 81,3 Not in Syllabus CBSE Exams 21 Ex 81,4 Important Not inQuestion what is the sum of the coefficients in the expansion of (ab)^5 Found 2 solutions by rfer, richard1234 Answer by rfer() (Show Source) You can put this solution on YOUR website!

A B 3 2 Expansion

Msbshse Solutions For Class 8 Maths Part 1 Chapter 5 Expansion Formulae Download For Free

So the answer is 3 3 3 × (3 2 × x) 3 × (x 2 × 3) x 3 (we are replacing a by 3 and b by x in the expansion of (a b) 3 above) Generally It is, of course, often impractical to write out Pascal"s triangle every time, when all that we need to know are the entries on the nth line Clearly, the first number on the nth line is 1 The1) State whether the following rational numbers will have terminating decimalexpansion or a nonterminating repeating expansion of decimal a) 17/8 Solution 17/8 = 17/(2 3 x 5 0) As the denominator is of the form 2 n 5 m so the expansion of decimal of 17/8 is terminating b) 64/255 solution 64/255 = 64/ (5 x 3 x 17) Clearly, 255 isIn mathematics, a trinomial expansion is the expansion of a power of a sum of three terms into monomialsThe expansion is given by ( ) = ∑ =,, (,,),where n is a nonnegative integer and the sum is taken over all combinations of nonnegative indices i, j, and k such that i j k = n The trinomial coefficients are given by (,,) =!!!!This formula is a special case of the multinomial

3 2 T Gpio Breakout Expansion Board 40pin Cable Diy Kit For Raspberry Pi B Business Industrial Electrical Equipment Supplies

A B 3 2 Expansion

Ss7c32 M The table below describes a type of government Executive Legislature Wthich title completes the table?= 1 and 0!= 5×4×3×2×1 = 1 and 8!

Http Www Maths Mq Edu Au Numeracy Web Mums Module2 Worksheet26 Module2 Pdf

Binomial Theorem Binomial Theorem Term 1 Unit 3 Ppt Video Online Download

What is the number of distinct terms in the expansion of (a b c) ?This means that the expansion of (ab)6 is (ab)6 = a6 6a5b15a4b2 a3b3 15a2b4 6ab5 b6 2 14 Factorial notation The factorial n!2B 3B 4 Channel RPi Relay Module Expansion Board for Raspberry Pi 3 2 A B 4 Channel RPi Relay Module Expansion Board for Raspberry Pi 3 2 A B 2B 3B NoteThe Raspberry Pi has 40Pin and 26Pin connectors This product is only compatible with the 40Pin Raspberry Pi Raspberry Pi GPIO interface For connecting Raspberry Pi

Solved In Exercises 17 Use The Column Row Expansion O Chegg Com

Misc 9 Expand Using Binomial Theorem 1 X 2 2 X 4 Miscellaneou

(x1)^2 7x (x3)^2 = 33 (x 5)(x 5) expand the brackets (x1)(x1) 7x (x3)(x3) = 33 (x5)(x5) multiply the brackets together using whichever method (i use the smiley face method) (x^2) 1 2x 7x (x^2) 9 6x = 33 (x^2) 25 collect the like terms either side of the equals sign (2x^2) 15x 10 = 33 (x^2) 25= (8 ⋅ 7 ⋅ 6 ⋅ 5)/ (4 ⋅ 3 ⋅ 2 ⋅ 1)(3x) 4 (2x 2 /3) 4 = 70(81x 4)(16x 8 /81) = 70(16)x 12 = 11 x 12 Example 2 Find the middle term in the expansion of (b/x x/b) 16 Solution Here n = 16, that is even So, theA^5b^5 Answer by richard1234(7193) (Show Source)

1 Leta Lind The Follwing A La 2 Use Expansion By Cofactors To Find The Determinant Of Homeworklib

X 2 X 3 Expand And Simplify Youtube

The calculator will find the binomial expansion of the given expression, with steps shown Show Instructions In general, you can skip the multiplication sign, so `5x` is equivalent to `5*x` In general, you can skip parentheses, but be very careful e^3x is `e^3x`, and e^(3x) is `e^(3x)`Find 31 ways to say expansion, along with antonyms, related words, and example sentences at Thesauruscom, the world's most trusted free thesaurusTo find an expansion for (a b) 8, we complete two more rows of Pascal's triangle Thus the expansion of is (a b) 8 = a 8 8a 7 b 28a 6 b 2 56a 5 b 3 70a 4 b 4 56a 3 b 5 28a 2 b 6 8ab 7 b 8 We can generalize our results as follows The Binomial Theorem Using Pascal's Triangle

Form 2 Unit 2 Lesson 5 Expansion Of Algebraic Expression Brilliant Maths

The Middle Term Of The Expansion Of 2x 2 3 3 2x 2 10 Is

N C 2 a n − 2 b 2 n C 1 a n − 1 b = n 3 C 3 a n 3 − 3 b 3 n 3 C 2 a n 3 − 2 b 2 In the expansion of (1 x) n by the increasing powers of x, the third term is four times as great as the fifth term, and the ratio of the fourth term to the sixth is 3 4 0Finding a Maclaurin Series

Expansion Of A Low Ms 7 2 5 2 B Middle Ms 3 2 1 2 And C Download Scientific Diagram

4 The Binomial Theorem

Amazon Com Makerfocus Raspberry Pi Expansion Board 4 Channel Relay Board Module Power Relay Module For Raspberry Pi 4b 3 Model B Raspberry Pi 3 2 Model B No Programming Required Programmable Computers Accessories

How To Expand Using The Identity A B 2 B2 2ab Youtube

3pcs 40pin T Type Gpio Adapter Expansion Board For Raspberry Pi 3 2 Model B B A Zero Sale Banggood Com Arrival Notice Arrival Notice

What Is A B 3 Quora

Coefficients A 1 2 And B 2a 3 2 In The Long Wave Expansion Equation 31 Download Scientific Diagram

Jee Mains 18 The Expansion Of 3x 2 1 2 Is Valid In Ascending Powers Of X If X Lies I Youtube

Gpio Multifunction Extended Expansion Board For Raspberry Pi B 3 2 Model B Business Industrial Electronic Components Semiconductors

The Binomial Expansion The Binomial Theorem On Vimeo

Binomial Theorem

8 Xx Xxx Math Resources For Taaleem Edison Schools

What Is The Formula Of Math A B 3 Math Quora

What Is The Formula Of Math A 3 B 3 Math Quora

11 1 Pascal S Triangle And The Binomial Theorem Ppt Download

Amazon Com Quickbuying 1pcs T Type Adapter Expansion Board For Raspberry Pi 3 2 Model B B A Zero For Bread Board Experiment Computers Accessories

What Is The Expansion Of A B C 3 Quora

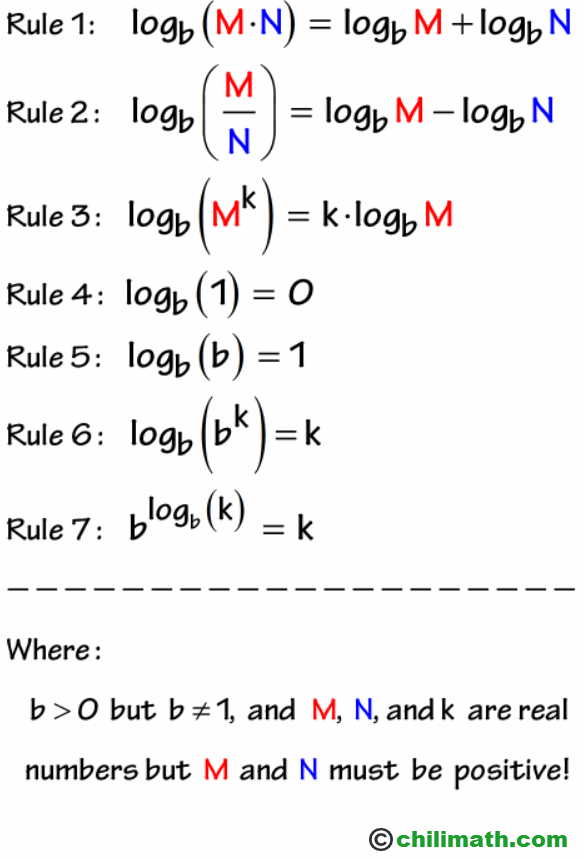
Logarithm Rules Chilimath

Ex 2 5 6 Write The Following Cubes In Expanded Form Ex 2 5

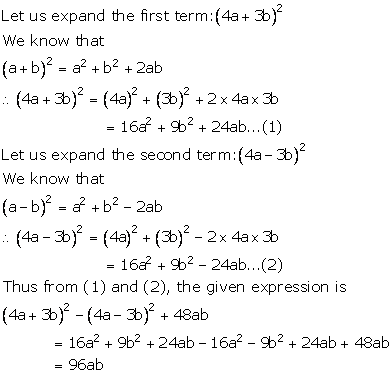
Chapter 4 Expansion Selina Concise Mathematics Part I Solutions For Class 9 Mathematics Icse Topperlearning

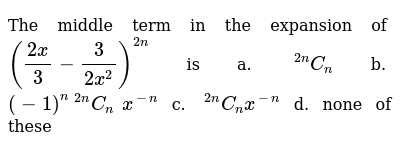
The Middle Term In The Expansion Of 2x 3 3 2x 2 2n Is A

2

Partial Fraction Expansion Video Khan Academy

Search Q A 5e3 2bb 5e3 Formula Tbm Isch

Expanded Form Ex The Latest Trend In Expanded Form Ex Expanded Form Writing Form

General And Middle Term Binomial Theorem Formula Videos Examples

9c2mat18 Algebra Expansion Ext

Madasmaths Com Archive Maths Booklets Standard Topics Various Binomial Series Expansions Exam Questions Pdf

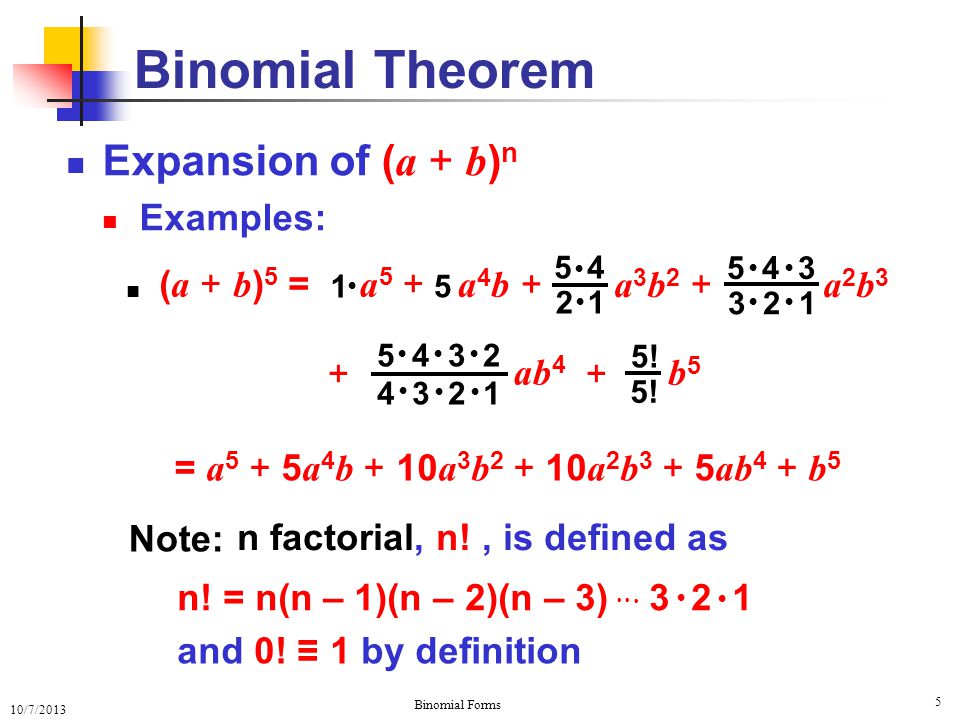
Binomial Forms Expansion Of Binomial Expressions Ppt Download

Combinations The Binomial Theorem The Standard Deck Of Playing Cards Consists Of 52 Cards Comprising Of Four Suits Clubs C Diamonds D Hearts H And Spades S Each Suit Has 13 Cards Ace A 2 3

Solved Find The Sixth Term Of The Binomial Expansion Course Hero

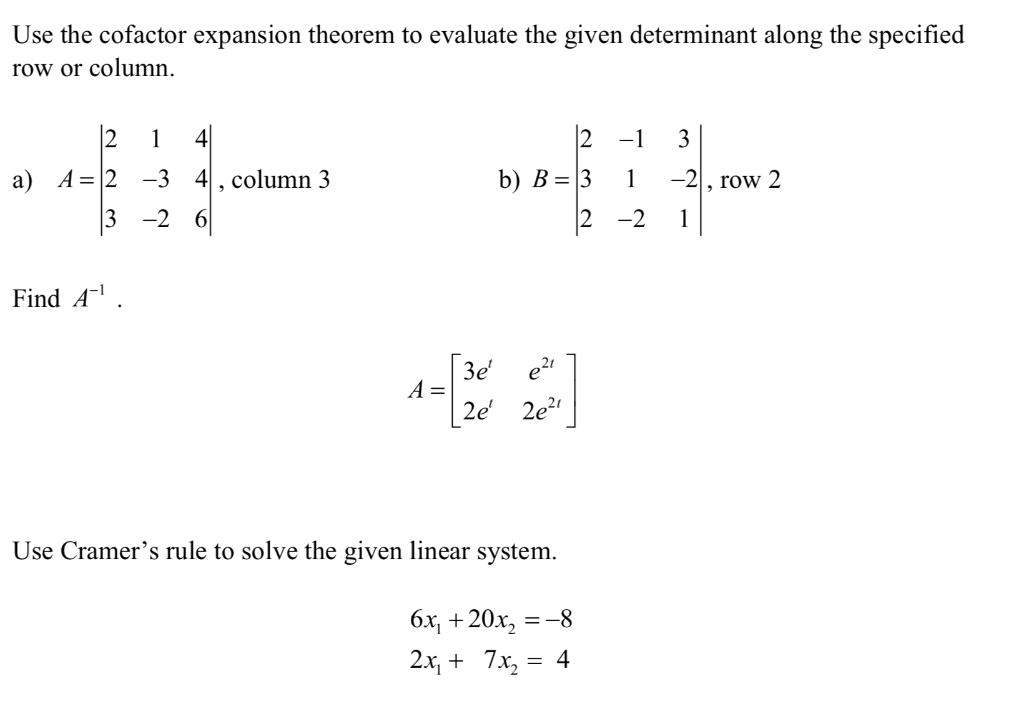
Solved Use The Cofactor Expansion Theorem To Evaluate The Chegg Com

A 2 B 2 Formula

Search Q A 5e2 2bb 5e2 Tbm Isch

Madasmaths Com Archive Maths Booklets Standard Topics Various Binomial Series Expansions Exam Questions Pdf

What Is The Expansion Of A B C 3 Quora

The Binomial Theorem

Need Help Asap Which Of The Following Is The Correct Expanded Form For The Series Below A 0 1 Brainly Com

Hdhdhd Rational Number Numbers

Math Help Algebra Quick Algebra Formulae Technical Tutoring

Expansion Tank Opel Frontera B 3 2 V6 Xdalys Lt

Gpio Multi Function Expansion Board For Raspberry Pi B 3 2 Model B Ebay

Wolfram Alpha Examples Step By Step Solutions

2

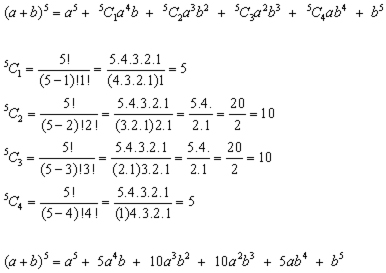
The Binomial Theorem Algebra Pure Mathematics From A Level Maths Tutor

Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9

Expanding Binomials Video Polynomials Khan Academy

Multi Function Extended Expansion Board Gpio For Raspberry Pi B 3 2 Model Ebay

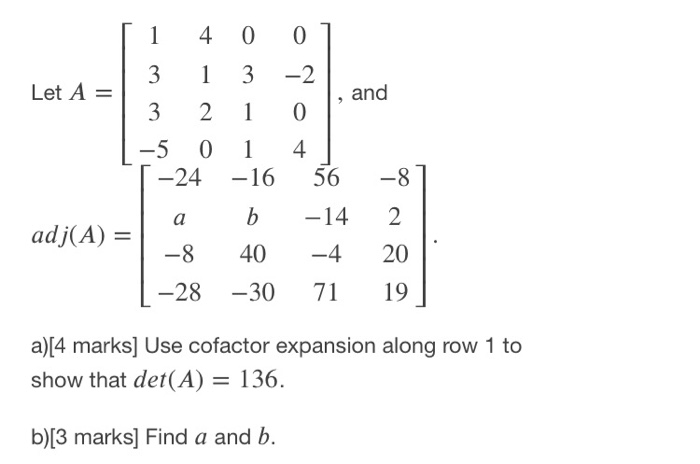
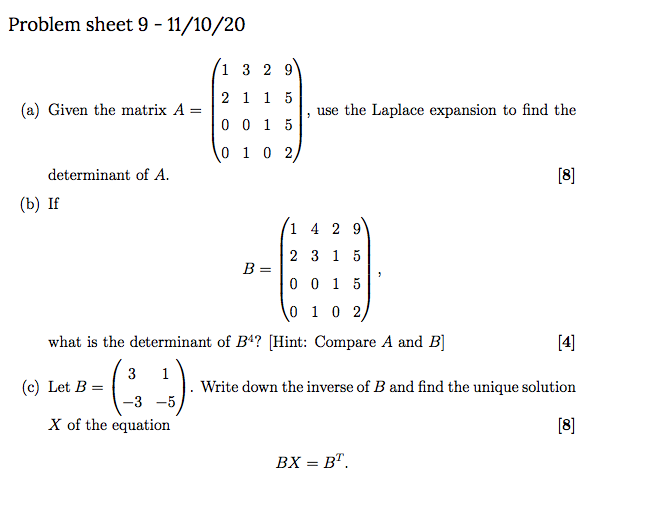
Solved 1 4 0 0 3 1 3 2 Let A And 0 3 2 5 0 24 1 1 1 Chegg Com

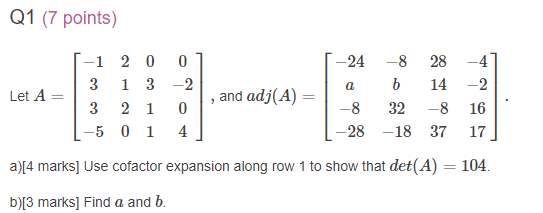
Solved Q1 7 Points Let A 1 2 0 0 3 1 3 2 3 2 1 0 5 0 Chegg Com

Chapter 4 Expansion Selina Concise Mathematics Part I Solutions For Class 9 Mathematics Icse Topperlearning

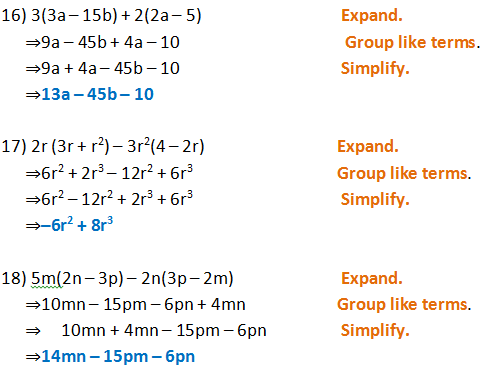
Form 2 Unit 2 Lesson 5 Expansion Of Algebraic Expression Brilliant Maths

Algebra Formulas A B 3 A B 2 A B C 3 A 3 B 3 Teachoo

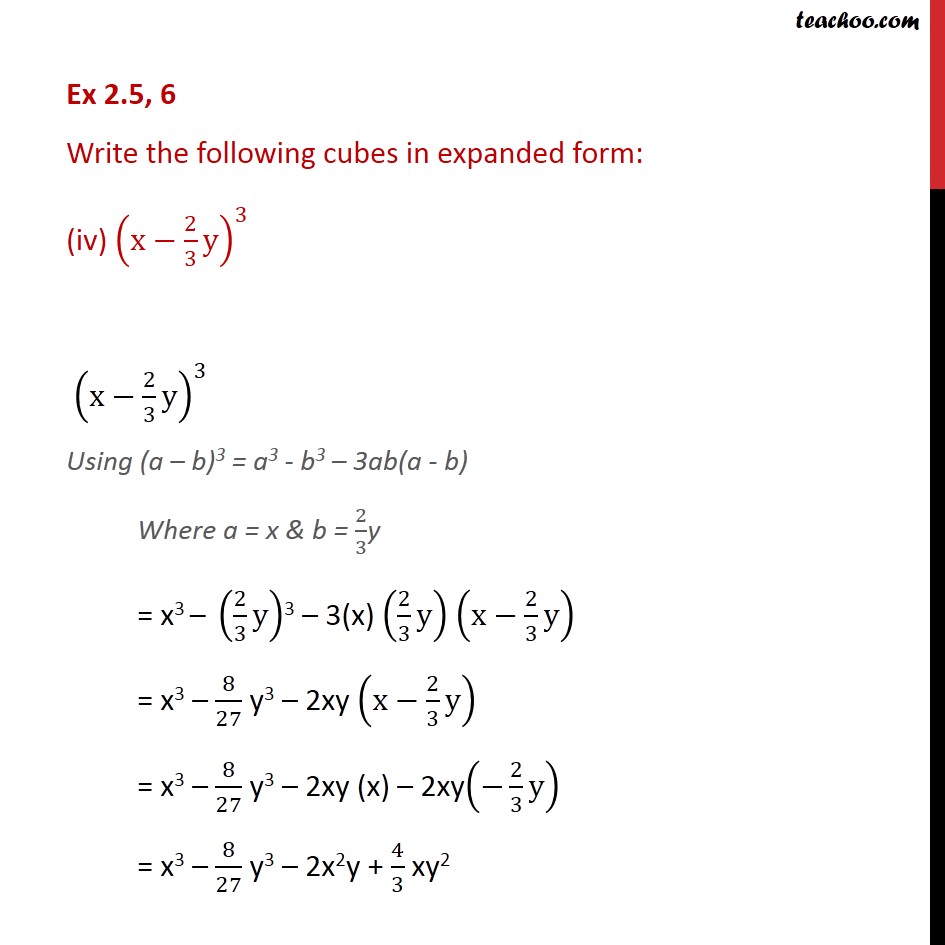
Ex 2 5 6 Write The Following Cubes In Expanded Form Ex 2 5

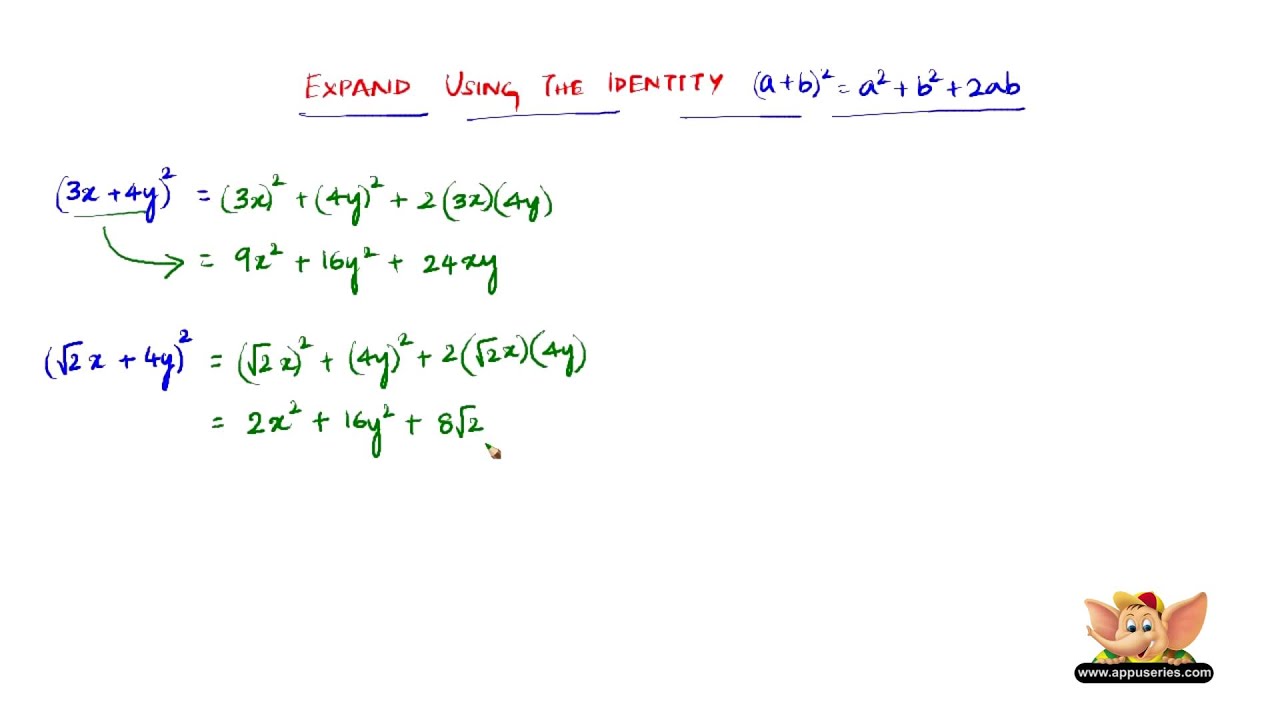
How To Expand Using The Identity A B 2 B2 2ab Youtube

Compute The Determinant Of The Matrix By Cofactor Expansion 3 2 5 1 1 4 3 Homeworklib

Misc 1 Find A B N In Expansion Of A B N If First Three

Amazon Com Xygstudy Arpi600 For Raspberry Pi 4 3 2 1 Model B B A Plus Supports Arduino Xbee Module With Various Interface Io Expansion Board Computers Accessories

The Binomial Theorem Fractional Powers Expanding 1 2x 1 3 Youtube

If T2 T3 In The Expansion Of A B N And T3 T4 In The A B N 3 Are Equal Then N

Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes

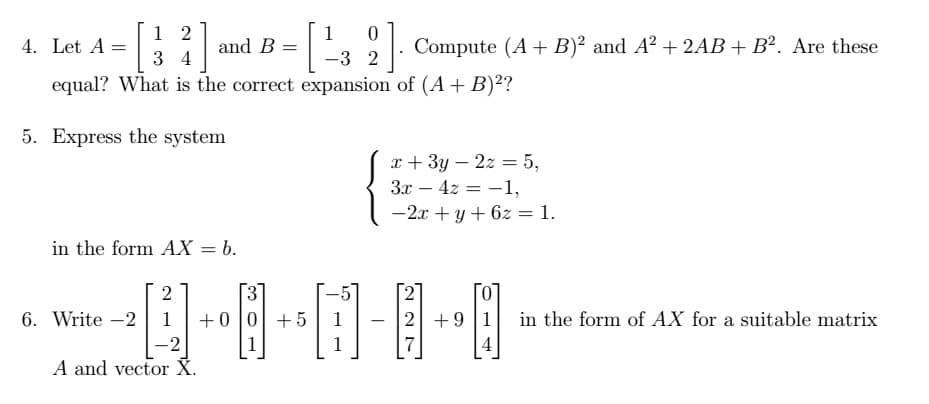
Solved 1 2 1 And B 0 Compute A B 2 And 2abb2 Are T Chegg Com

How Do I Use The Binomial Theorem To Find The Constant Term Socratic

Evaluate Each Statement For P 5 Once Before You Expand It And One After You Expand It A 4 3p 2 B 3p 6 P C Math 2p 2 4p 3 Math D Math P 3p 2 4p 4 Math E Explain

What Is The Formula Of Math A B 3 Math Quora

Gpio Multifunction Extended Expansion Board For Raspberry Pi B 3 2 Model B Business Industrial Electronic Components Semiconductors

Q Tbn And9gcrswdldblxmiitn Ggstx6knbcgxw3sex0r673zeswoh Igfkvn Usqp Cau

Binomial Theorem Properties Terms In Binomial Expansion Examples Pdf

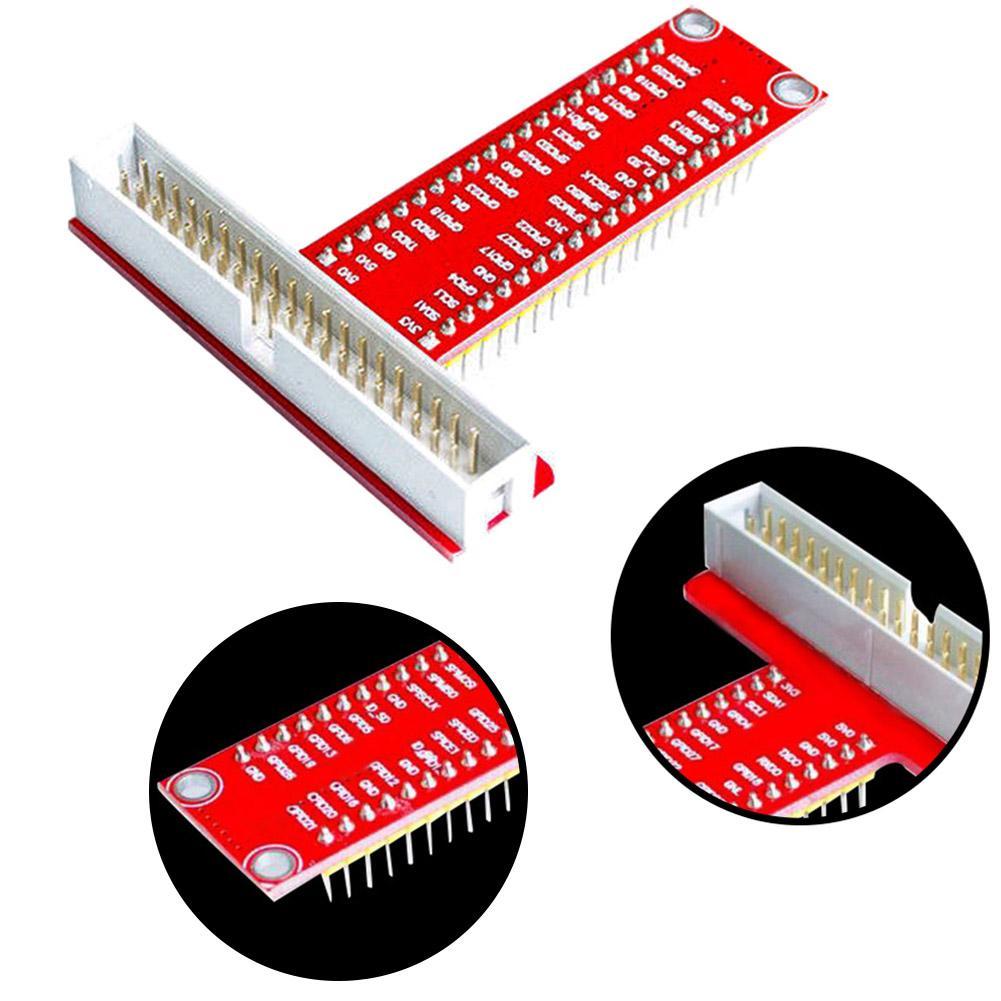
Electrical Equipment Supplies 40pin Cable Diy Kit For Raspberry Pi B 3 2 T Gpio Breakout Expansion Board Business Office Industrial Saintdi Com

T Gpio Breakout Expansion Board 3 2 40pin Cable Diy Kit For Raspberry Pi B Electrical Equipment Supplies Other Electrical Equipment Supplies

Answered 16 Find The Coefficient Of X In The Bartleby

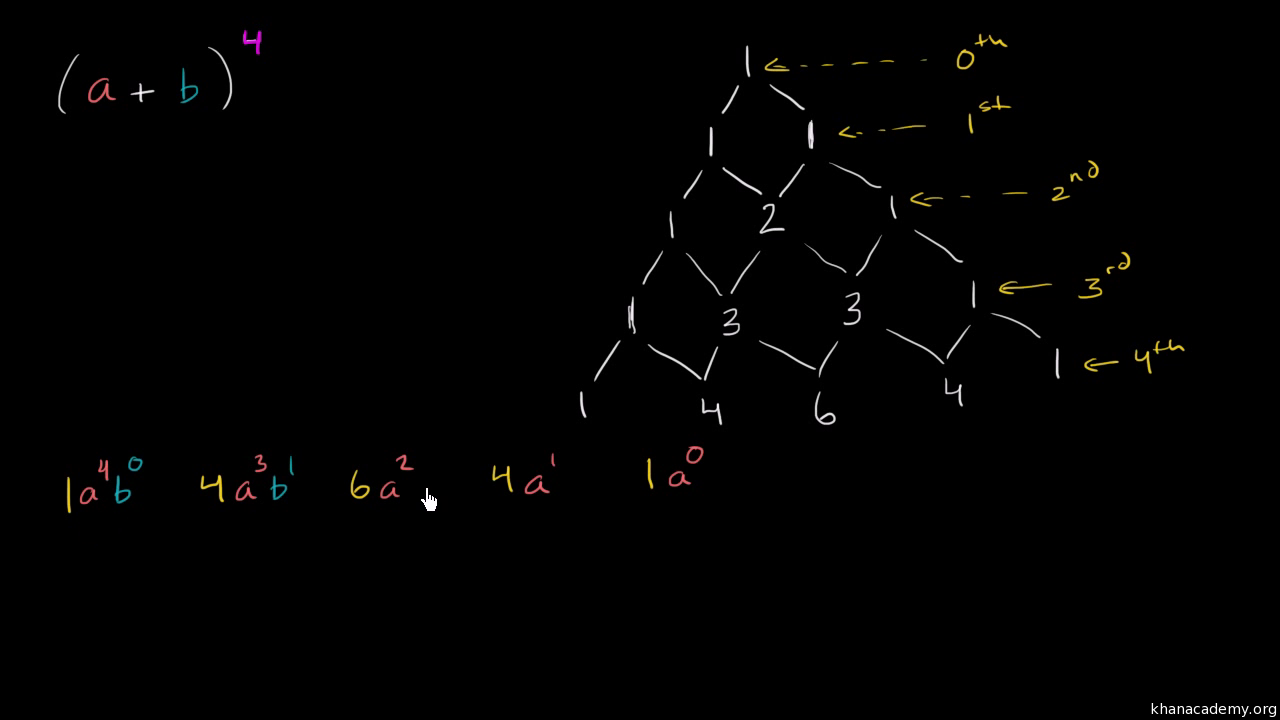
Pascal S Triangle And Binomial Expansion Video Khan Academy

Incoming Term: (a+b)^3/2 expansion,